Teaching rigid transformations in geometry units can be so much fun! It's different from many other topics that we teach and there are so many ways to connect rigid transformations to the real world and use hands on activities. On the other hand, sometimes students just need lots and lots of practice to master a new concept. Let's look at the three types of rigid transformations taught in middle school math and geometry classes.
Reflections, Rotations, and Translations - Rigid Transformations
1. What is a reflection? It's a mirror image of a shape, usually across an axis or a line of reflection.
One fun way to introduce reflections is with mirrors. Students usually have prior knowledge of reflections from their own experiences.
The trickiest part of reflections for students is flipping the shape over the correct axis. They need to remember that the x-axis is the horizontal line and the y-axis is the vertical line. Both axes meet at the origin, which is the point (0, 0).
2. What is a rotation? It's turning a shape or line around a point, called the center of rotation.
Think about a basketball player pivoting off of one foot before making a pass to another player.
A great way to introduce degrees of rotation is to practice it with the class standing up and turning clockwise or counter-clockwise any number of degrees.
You can call out "90 degrees clockwise" and watch the students make a quarter turn. Then call out "180 degrees" and discuss why is doesn't matter if they turn clockwise or counter-clockwise for that problem.
Finally, discuss how 270 degrees clockwise would be the same as a 90 degree counter-clockwise turn.
Before playing this game, make sure to show slides or draw on the board a coordinate plane or clock and discuss each vocabulary word with visual aids.
Once students are ready to draw rotations on coordinate planes, use patty paper, or tracing paper, to draw the original and then rotate the paper to see where the new shape will end up.
3. What is a translation? It's sliding a shape in a straight line without turning or flipping it.
Students usually find translations the easiest, since the shape will remain facing the same direction.
Practice translations up and down, and then left and right, before combining the directions. For example, a problem might ask students to "translate left 3 units," or "translate down 2 units." Then, a more complicated problem would ask students to "translate up 5 units and right 4 units."
Sequences of Rigid Transformations in Geometry
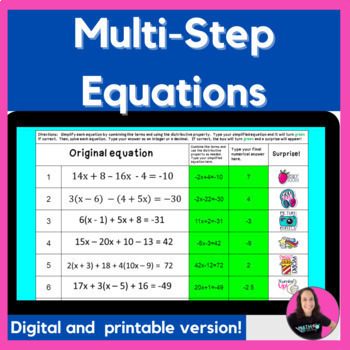
Once students have mastered each transformation separately, it's time to work on sequences. A sequence of transformations is more than one transformation at a time.
For example, a student may be asked to "translate figure A 5 units down and reflect over the y-axis."
Students will need a lot of practice of the individual transformations and the sequences of transformations.
Isn't a Dilation a Transformation, too?
Yes, a dilation is a transformation, but it is not a rigid transformation.
A rigid transformation means that a figure will stay the same size and the same shape.
If a dilation is performed, then the shape will get bigger or smaller by a scale factor.
If the scale factor is greater than 1, the figure will be enlarged. If the scale factor is smaller than 1 but greater than 0, then the figure will be reduced.
Rigid Transformations - In Conclusion
To sum up, don't be afraid to try a lot of different activities with transformations to help your students understand each one and practice these skills.
Need a whole unit of activities for transformations?